Above we considered three different spectral units: frequency, ν, (Hz), wavelength, λ, (μm) and wavenumber, σ, (cm-1). We derived expressions for the spectral radiances Lν , Lλ , and Lσ . To find the radiance, L (W m-2 sr-1), we can integrate any of these over the respective spectral
variable. That is,
We will perform the integration of Lν over all frequencies, ν :
(Here we used the result Σ n-4 = ζ (4) = π 4/90, where ζ is the Reimann zeta function) The total radiated power per unit
area, called the radiant emittance or radiant exitance, M, can be found by further integrating with respect to solid angle over the
hemisphere into which the surface radiates. A source whose radiance is
independent of angle is called Lambertian (from Lambert’s cosine law of reflection). This is implicitly assumed
for an ideal blackbody, and is a good approximation for many real
sources. For a Lambertian source, M is related to L by
so
This is the Stefan-Boltzmann law, and the quantity in parentheses is the
Stefan-Boltzmann constant. A common mistake in deriving this result is to
assume the factor is 2π rather than π, because there are 2πsteradians in the hemisphere, but this
neglects the cosθ reduction from Lambert’s cosine law.
Similarly, the total photon radiance is found by integrating LσP over all frequencies:
Recall ζ is the Riemann zeta function. ζ(3) ≈ 1.202056903159594 is also known as Apéry’s constant.
Integrating LσP over the hemisphere (again assuming a
Lambertian source) gives
This is the Stefan Boltzmann law for photon radiant emittance. Notice
that the total photon flux is proportional to T 3, whereas the total
power, given by (5), is proportional to T 4.














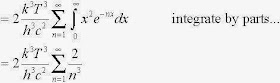




Tidak ada komentar:
Posting Komentar